(Figure 1)

PENTOMINO WOOD MOSAICS
(Excerpt from Guenter Albrecht-Buehler: 'The Pentomino Workshop' (German) Fischer Taschenbuch Verlag, Frankfurt (1992))
This is about pentominoes. In case you have not yet encountered them, they are flat shapes formed from five (Gr.pente = five) unit squares. The squares must touch each other along an edge, but not merely at a corner. For example, the shape shown in Figure 1 a is a pentomino, whereas the one in figure 1b is not.
(Figure 1)

( Please note, the present article considers only pentomino arrangements in a plane. However, pentominoes can also acquire a three-dimensional aspect if one makes each of the flat shapes 1 unit thick. My pentomino wood mosaics show a great many three-dimensional shapes in perspective which are constructed from pentominoes. For some other specific and intriguing three-dimensional problems about pentominoes see also Arlet Ottens' Pentomino-problem).
In addition to pentominoes there are also so-called 'pentacubes'. These are the 29 truely three-dimensional shapes formed from 5 cubes that touch each other on at least one face. ( For refences see: Martin Gardner, "Knotted Doughnuts", W. H. Freeman & Co, New York [1986], Chapter 3 ff.).
Not counting mirror images of pentominoes or the shapes that result from rotating one by 90 degrees, there are exactly 12 different shapes. They are often named by the capital letter of the alphabet they resemble most.
(Figure 2 )

If you count reflections and rotations, there are 63 shapes: 8 possibilities of rotation and reflection for the asymmetrical pentominoes F, L, N, P, and Y; 4 possibilities for the mirror- or point-symmetrical pentominoes T, U, V, W, and Z; 2 possibilities for the I and 1 for the completely symmetrical X.
Pentominoes are the brainchilds of the 22 year old graduate student of mathematics at Harvard, Solomon W. Golomb.In 1954 he studied the general shapes one can form from N unit squares. If N = 1 there is only one shape, namely the monomino. Similarly, for N = 2, only the domino exists. Combining 3 squares gives rise to the 2 triominoes. N = 4 yields 5 tetrominoes. For N = 5 we arrive at the 12 pentominoes. There are 35 hexominoes, and so forth. (There is a program by Ambros Marzetta to compute the different polyominoes of rank N ).
It seems, Solomon Golomb was quite aware of the potential of his 'polyominoes', as he called them, and he presented them to American puzzle fans at the Harvard Mathematics Club. Yet, no major 'movement' followed, until Martin Gardner, the great mathematical brainteaser from SCIENTIFIC AMERICAN found out about pentominoes, and told his readership about them. Soon all over the world, people began to cut the shapes out of paper and to play with these pieces of an entirely novel jig-saw puzzle. The small number of single monominos and dominos, the 2 triominoes and 5 tetrominoes turned out to be somewhat uninspiring because their possibilities seemed quickly exhausted. The 35 hexominoes and the 108 heptominoes were too numerous and too diverse to allow solutions of polyomino problems to be elegant. However, the 12 pentominoes were complex enough to pose tantalizing problems while their small number of twelve permitted mathematically elegant and graphically pleasing solutions.
The 'movement' generated much excitement. In 1965, Solomon W. Golomb's book 'Polyominoes' (Charles Scribner's Sons, New York, 1959) appeared. Martin Gardner included several chapters on pentominoes in his books such as 'Mathematical Puzzles and Diversions ' (Simon and Schuster, New York, 1959). Most people recovered soon after, others including me never lost their addiction.
The pentominoes are not only pieces of a special jig-saw puzzle, but an alphabet for the composition of logically complete texts that can express ambivalence, paradox and infinity. In contrast to all other literary, musical or graphical alphabets, the alphabet of the pentominoes is complete: They are the only and all the variations on the theme of five squares. There is a complete inner logic to them. Indeed, they arise from each other by moving one of the five squares to another place in a never-ending circle.
(Figure 3).

They express infinity. Each pentomino can be composed of nine different pentominoes made of three times smaller squares. Each of the smaller pentominoes can be composed of nine even smaller ones. And so forth: Infinite regress, fractals arise.
(Figure 4).

They express simplicity. The twelve pentominoes can be arranged to fill simpler shapes, namely rectangles of the area of 5 x 12 = 60 unit squares. For example, rectangles with the sides (6 x 10), (5 x 12), (4 x 15), (3 x20) can be composed of the twelve fundamental shapes.
(Figure 5).
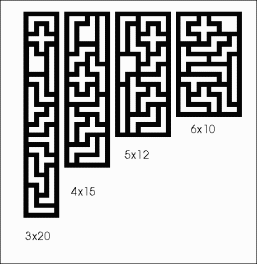
They generate ambiguity. There is not only one way to form rectangles from the 12 pentominoes. Among the trillions of possibilities to arrange the twelve pentominoes in a 6x10 rectangle, there are in excess of 2000 'solutions' which fill the rectangle exactly. According to Arthur C. Clarke's 'Imperial Earth' there are 2,339 solutions. Recently, I wrote a computer program to convince myself that the number is correct. (You can download a copy and run it in DOS together with the complete listing of the 6x10 solutions). Each solution has its own fascinating peculiarities, symmetries, and other esthetic qualities. After several days of trying in vain to find a solution, the pentomino novice may not believe that there is even one. In order to be a bit more precise, the odds to find by chance a solution of the problem to fill a 6x10 rectangle with the 12 pentominoes is very, very small because there are there are 2000+ solutions and N = 12!x85x45x2= 3x1016 ways to place the 12 pentominoes in their various orientations inside the rectangle.
They express complementary opposites. It is possible to form a Yin-Yang symbol from the 12 pentominoes.
(Figure 6).
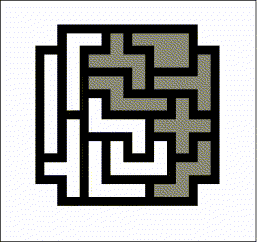
Furthermore, in an Escherian manner one can make the interspace between 6 pentominoes become the other 6 pentominoes. The figure shows an example where the space between F and X becomes W.
(Figure 7).

These and many more surprising qualities of pentominoes make them candidates for artistic expression. Indeed, in the original version of the film '2001, A Space Odyssey' Arthur C. Clarke, a pentomino addict himself, had the HAL Computer play pentomino games against astronaut Bowman. Later, in his 'Imperial Earth', Arthur C. Clarke devoted a chapter to the pentominoes. Thus they entered the literary world.
The present collection of wood mosaics tries to bring them closer to the visual arts. The pentominoes, cut out of various veneers, are based on unit squares with 1 inch sides, because the length of 1 inch or integral multiples or fractions thereof seem to agree particularly well with our sense of proportions. The pieces were arranged on a 'unit grid' with sides of 32 and 48 inches, which come close to the famous 'golden ratio' 2/(SQRT(5)-1) ) that plays an important role in visual composition. Each of the mosaics presents variations on a particular theme and solutions to a particular problem about pentominoes under the geometric constraints of the unit grid. The ordered, yet living texture of wood veneers was chosen for the surface of pentominoes, because it offered the expression of mathematical accuracy while reducing the impersonal coldness of overly cerebral graphics. The limited range of colors of wood was intended to focus the viewer's attention on the importance of the shapes without dazzling the eye with colors.
The possibilities to make such panels are difficult to exhaust in your or my lifetime. Each panel has 32x48 = 1536 squares, which allows to place 1536/5 = 307 pentomino shapes. On average, each has 63/12 = 5 different orientations. Hence, there are (12x5)307 possibilities which is approximately 10533, not counting, that each of the shapes can be cut from a vast variety of differently colored wood veneers. Considering that each panel takes a month's work and that the universe is about 1011 months old, we still have quite a few ages of the universe to go before we run out of possibilities to compose pentomino wood mosaics.
As you look at the abstract patterns around you on the partitioning walls of the Fermilab art gallery two major questions may come to mind:
1. What do they mean?
and
2. Now that we know that Albrecht-Buehler can make abstract pictures, can he also draw a horse?
As to the first question about their meaning, they are intended as exercises in the different ways to compose patterns of pentominoes.
As to the second question, no, I cannot draw a horse. My children are much more talented, and consequently, in our family they are in charge of drawing horses. Why then would I dare to show you these panels?
I have asked myself this question quite often. In fact, I have worked in total secrecy, too embarrassed about my lack of graphic horsemanship to show the results to anyone. Yet, quite recently, I discovered the answer to my problem: I am not a visual artist!
Obviously, many people could have told me that. In fact for years I hid my activities in fear that they would do so. However this time I realized that nobody asks a writer or composer to draw horses as proof of qualification. Well, as I pointed out earlier, pentominoes are an alphabet, and I arrange them on a unit grid much in the way of a composer or writer. Why should I be able to draw horses?
Having cleared that out of the way, let me thank you again and express my sincere hope that you all become pentomino addicts.
(Visit also Ashish Mahabal's page.)